Class 10 – Introduction to Trigonometry – Previous years Questions
Previous Years Questions Important QuestionsIntroduction to Trigonometry – Previous years Questions
- The value of θ for which cos(10° + θ) = sin 30° , is
- 50°
- 40°
- 80°
- 20° [CBSE 2020] [1 Mark]
- The value of θ for which sin (44° + θ) = cos 30° , is
- 46°
- 60°
- 16°
- 90° [CBSE 2020] [1 Mark]
- If tan A = 1, then 2 sin A cos A = ________. [CBSE 2020] [1 Mark]
- What is the value of (cos2 67° – sin2 23°)? [CBSE 2018] [1 Mark]
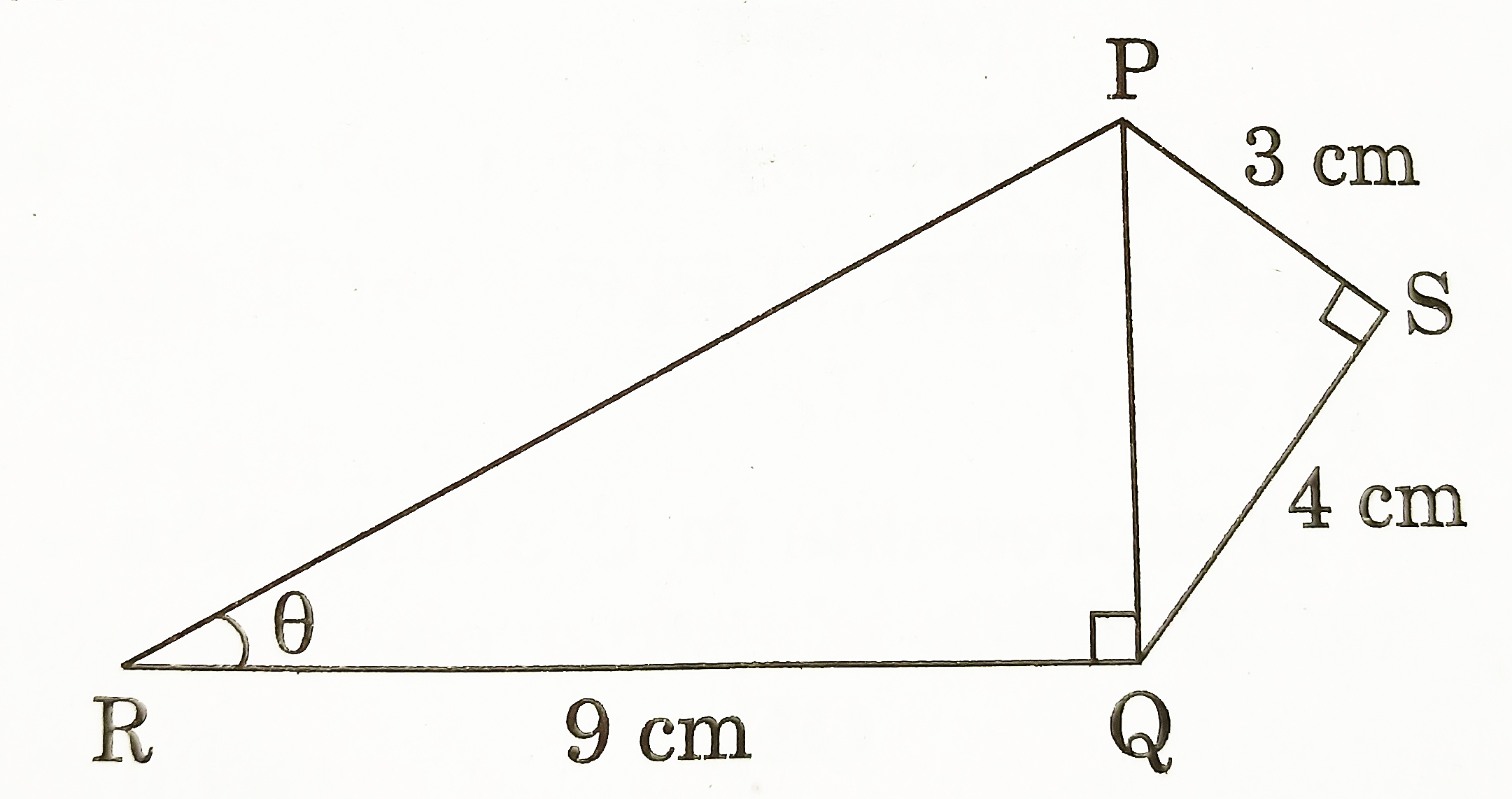
- In figure, PS = 3 cm, QS = 4 cm, ∠PRQ = θ, ∠PSQ = 90° , PQ ⊥ RQ and RQ = 9 cm. Evaluate tan θ. [CBSE 2019] [1 Mark]
- If tan α = 5/12, find the value of sec α. [CBSE 2019] [1 Mark]
- Evaluate:
[CBSE 2020] [2 Marks]
- Evaluate:
[CBSE 2020] [2 Marks]
- If 4 tan θ = 3, evaluate
. [CBSE 2018] [3 Marks]
- If tan 2A = cot(A -18°), where 2A is an acute angle, find the value of A. [CBSE 2018] [3 Marks]
- A, B and C are interior angles of a triangle ABC. Show that
- If ∠A = 90°, then find the value of tan
. [CBSE 2019] [3 Marks]
- If tan (A+B) = 1 and tan (A-B) = 1/√3, 0° < A+B < 90°, A>B, then find the value of A and B. [CBSE 2019] [3 Marks]
- Prove that :
[CBSE 2020] [3 Marks]
- Prove that
. [CBSE 2018] [4 Marks]
- If 1+sin2 θ = 3sin θ cos θ, then prove that tan θ = 1 or tan θ = 1/2. [CBSE 2019] [4 Marks]
- Prove the following:
[CBSE 2019] [4 Marks]
- Prove that:
[CBSE 2019] [4 Marks]


Comments
Sudhanshu Raj
sir answer to likh dejiye